Journal of Integer Sequences, Vol. 17 (2014), Article 14.9.1

A Famous Identity of Hajós in Terms of Sets


Rui Duarte
Center for Research and Development in Mathematics and Applications
Department of Mathematics
University of Aveiro
3810-193 Aveiro
Portugal

António Guedes de Oliveira
CMUP and Mathematics Department
Faculty of Sciences
University of Porto
4169-007 Porto
Portugal

Abstract:

By considering the famous identity on the convolution of the central binomial coefficients

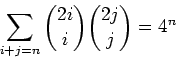
\begin{displaymath}\sum_{i+j=n} {{2i} \choose i} {{2j} \choose j} = 4^n\end{displaymath}

in terms of pairs of $\ell$-subsets of $2\ell$-sets, we obtain a new bijective proof and new identities that can be seen as refinements.
Full version:  pdf,    dvi,    ps,    latex    

(Concerned with sequences A051288 A085841 A089627 A105070 A229032.)

Received September 11 2013; revised version received June 6 2014; August 7 2014. Published in Journal of Integer Sequences, August 12 2014.

Return to Journal of Integer Sequences home page