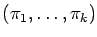
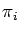
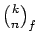
An integer composition of a nonnegative integer n is a tuple of nonnegative integers whose sum is n; the 's are called the parts of the composition. For fixed number k of parts, the number of f-weighted integer compositions (also called f-colored integer compositions in the literature), in which each part size s may occur in f(s) different colors, is given by the extended binomial coefficient . 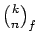We derive several congruence properties for , most of which are analogous to those for ordinary binomial coefficients. 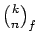Among them is the parity of , Babbage's congruence, Lucas' theorem, etc. We also give congruences for c_f(n), the number of f-weighted integer compositions with arbitrarily many parts, and for extended binomial coefficient sums. We close with an application of our results to prime criteria for weighted integer compositions.