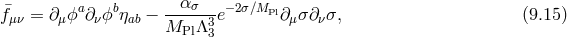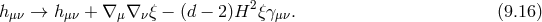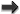9 Extensions of Ghost-free Massive Gravity
Massive gravity can be seen as a theory of a spin-2 field with the following free parameters in addition to the standard parameters of GR (e.g., the cosmological constant, etc…),- Reference metric
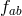 ,
,
- Graviton mass
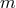 ,
,
 dimensionless parameters
dimensionless parameters 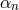 (or the
(or the 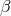 ’s) .
’s) .
As natural extensions of massive gravity one can make any of these parameters dynamical. As already seen, the reference metric can be made dynamical leading to bi-gravity which in addition to massive spin-2 field carries a massless one as well.
Another natural extension is to promote the graviton mass 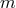 , or any of the free parameters
, or any of the free parameters 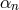 (or
(or
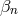 ) to a function of a new dynamical variable, say of an additional scalar field
) to a function of a new dynamical variable, say of an additional scalar field 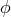 . In principle the mass
. In principle the mass
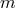 and the parameters
and the parameters  ’s can be thought as potentials for an arbitrary number of scalar fields
’s can be thought as potentials for an arbitrary number of scalar fields
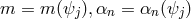 , and not necessarily the same fields for each one of them [320*]. So long as these
functions are pure potentials and hide no kinetic terms for any new degree of freedom, the constraint
analysis performed in Section 7 will go relatively unaffected, and the theory remains free from the BD
ghost. This was shown explicitly for the mass-varying theory [319*, 315*] (where the mass is promoted to
a scalar function of a new single scalar field,
, and not necessarily the same fields for each one of them [320*]. So long as these
functions are pure potentials and hide no kinetic terms for any new degree of freedom, the constraint
analysis performed in Section 7 will go relatively unaffected, and the theory remains free from the BD
ghost. This was shown explicitly for the mass-varying theory [319*, 315*] (where the mass is promoted to
a scalar function of a new single scalar field, 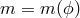 , while the parameters
, while the parameters 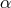 remain
constant23),
as well as a general massive scalar-tensor theory [320*], and for quasi-dilaton which allow for different
couplings between the spin-2 and the scalar field, motivated by scale invariance. We review these models
below in Sections 9.1 and 9.2.
remain
constant23),
as well as a general massive scalar-tensor theory [320*], and for quasi-dilaton which allow for different
couplings between the spin-2 and the scalar field, motivated by scale invariance. We review these models
below in Sections 9.1 and 9.2.
Alternatively, rather than considering the parameters 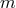 and
and 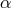 as arbitrary, one may set them to
special values of special interest depending on the reference metric
as arbitrary, one may set them to
special values of special interest depending on the reference metric 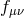 . Rather than an ‘extension’ per se
this is more special cases in the parameter space. The first obvious one is
. Rather than an ‘extension’ per se
this is more special cases in the parameter space. The first obvious one is 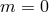 (for arbitrary reference
metric and parameters
(for arbitrary reference
metric and parameters 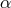 ), for which one recovers the theory of GR (so long as the spin-2
field couples to matter in a covariant way to start with). Alternatively, one may also sit on
the Higuchi bound, (see Section 8.3.6) with the parameters
), for which one recovers the theory of GR (so long as the spin-2
field couples to matter in a covariant way to start with). Alternatively, one may also sit on
the Higuchi bound, (see Section 8.3.6) with the parameters  ,
, 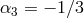 and
and
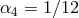 in four dimensions. This corresponds to the partially massless theory of gravity,
which at the moment is pathological in its simplest realization and will be reviewed below in
Section 9.3.
in four dimensions. This corresponds to the partially massless theory of gravity,
which at the moment is pathological in its simplest realization and will be reviewed below in
Section 9.3.
The coupling massive gravity to a DBI Galileon [157*] was considered in [237*, 461, 261] leading to a generalized Galileon theory which maintains a Galileon symmetry on curved backgrounds. This theory was shown to be free of any Ostrogradsky ghost in [19*] and the cosmology was recently studied in [315*] and perturbations in [20*].
Finally, as other extensions to massive gravity, one can also consider all the extensions applicable to GR.
This includes the higher order Lovelock invariants in dimensions greater than four, as well as promoting the
Einstein–Hilbert kinetic term to a function 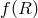 , which is equivalent to gravity with a scalar field. In the
case of massive gravity this has been performed in [89*] (see also [46, 354]), where the absence of BD ghost
was proven via a constraint analysis, and the cosmology was explored (this was also discussed in
Section 5.6 and see also Section 12.5).
, which is equivalent to gravity with a scalar field. In the
case of massive gravity this has been performed in [89*] (see also [46, 354]), where the absence of BD ghost
was proven via a constraint analysis, and the cosmology was explored (this was also discussed in
Section 5.6 and see also Section 12.5). 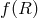 extensions to bi-gravity were also derived in
[416*, 415*].
extensions to bi-gravity were also derived in
[416*, 415*].
Trace-anomaly driven inflation in bi-gravity was also explored in Ref. [47]. Massless quantum effects can
be taking into account by including the trace anomaly 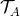 given as [203]
given as [203]
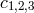 are three constants depending on the field content (for instance the number of scalars,
spinors, vectors, graviton etc.) Including this trace anomaly to the bi-gravity de Sitter-like solutions were
found which could represent a good model for anomaly-driven models of inflation.
are three constants depending on the field content (for instance the number of scalars,
spinors, vectors, graviton etc.) Including this trace anomaly to the bi-gravity de Sitter-like solutions were
found which could represent a good model for anomaly-driven models of inflation.
9.1 Mass-varying
The idea behind mass-varying gravity is to promote the graviton mass to a potential for an external scalar
field 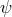 ,
, 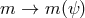 , which has its own dynamics [319*], so that in four dimensions, the dRGT action for
massive gravity gets promoted to
, which has its own dynamics [319*], so that in four dimensions, the dRGT action for
massive gravity gets promoted to
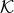 are given in (6.7*). This could also be performed for bi-gravity, where we would simply
include the Einstein–Hilbert term for the metric
are given in (6.7*). This could also be performed for bi-gravity, where we would simply
include the Einstein–Hilbert term for the metric 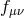 . This formulation was then promoted not only to
varying parameters
. This formulation was then promoted not only to
varying parameters 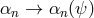 but also to multiple fields
but also to multiple fields  , with
, with 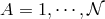 in [320*],
The absence of BD ghost in these theories were performed in [319*] and [320*] in unitary gauge, in the ADM
language by means of a constraint analysis as formulated in Section 7.1. We recall that in the
absence of the scalar field
in [320*],
The absence of BD ghost in these theories were performed in [319*] and [320*] in unitary gauge, in the ADM
language by means of a constraint analysis as formulated in Section 7.1. We recall that in the
absence of the scalar field 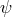 , the primary second-class (Hamiltonian) constraint is given by
In the case of a mass-varying theory of gravity, the entire argument remains the same, with the simple
addition of the scalar field contribution,
where
, the primary second-class (Hamiltonian) constraint is given by
In the case of a mass-varying theory of gravity, the entire argument remains the same, with the simple
addition of the scalar field contribution,
where 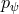 is the conjugate momentum associated with the scalar field
is the conjugate momentum associated with the scalar field 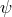 and
Then the time-evolution of this primary constraint leads to a secondary constraint similarly as in
Section 7.1. The expression for this secondary constraint is the same as in (7.33*) with a benign new
contribution from the scalar field [319]
Then as in the normal fixed-mass case, the tertiary constraint is a constraint for the lapse and the system of
constraint truncates leading to 5+1 physical degrees of freedom in four dimensions. The same logic goes
through for generalized massive gravity as explained in [320*].
and
Then the time-evolution of this primary constraint leads to a secondary constraint similarly as in
Section 7.1. The expression for this secondary constraint is the same as in (7.33*) with a benign new
contribution from the scalar field [319]
Then as in the normal fixed-mass case, the tertiary constraint is a constraint for the lapse and the system of
constraint truncates leading to 5+1 physical degrees of freedom in four dimensions. The same logic goes
through for generalized massive gravity as explained in [320*].
One of the important aspects of a mass-varying theory of massive gravity is that it allows more flexibility for the graviton mass. In the past the mass could have been much larger and could have lead to potential interesting features, be it for inflation (see for instance Refs. [315*, 378*] and [282*]), the Hartle–Hawking no-boundary proposal [498*, 439*, 499*], or to avoid the Higuchi bound [307*], and yet be compatible with current bounds on the graviton mass. If the graviton mass is an effective description from higher dimensions it is also quite natural to imagine that the graviton mass would depend on some moduli.
9.2 Quasi-dilaton
The Planck scale 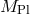 , or Newton constant explicitly breaks scale invariance, but one can easily
extend the theory of GR to a scale invariant one
, or Newton constant explicitly breaks scale invariance, but one can easily
extend the theory of GR to a scale invariant one 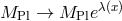 by including a dilaton scalar
field
by including a dilaton scalar
field 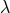 which naturally arises from string theory or from extra dimension compactification
(see for instance [122] and see Refs. [429, 120, 248] for the role of a dilaton scalar field on
cosmology).
which naturally arises from string theory or from extra dimension compactification
(see for instance [122] and see Refs. [429, 120, 248] for the role of a dilaton scalar field on
cosmology).
When dealing with multi-gravity, one can extend the notion of conformal transformation to the global
rescaling of the coordinate system of one metric with respect to that of another metric. In the case of
massive gravity this amounts to considering the global rescaling of the reference coordinates with respect to
the physical one. As already seen, the reference metric can be promoted to a tensor with respect to
transformations of the physical metric coordinates, by introducing four Stückelberg fields  ,
,
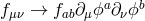 . Thus the theory can be made invariant under global rescaling of the reference
metric if the reference metric is promoted to a function of the quasi-dilaton scalar field
. Thus the theory can be made invariant under global rescaling of the reference
metric if the reference metric is promoted to a function of the quasi-dilaton scalar field  ,
,
Recently, the decoupling limit of the original quasi-dilaton model was derived in [239]. Interestingly, a new self-accelerating solution was found in this model which admits no instability and all the modes are (sub)luminal for a given realistic set of parameters. The extension of this solution to the full theory (beyond the decoupling limit) should provide for a consistent self-accelerating solution which is guaranteed to be stable (or with a harmless instability time scale of the order of the age of the Universe at least).
9.2.1 Theory
As already mentioned, the idea behind quasi-dilaton massive gravity (QMG) is to extend massive gravity to
a theory which admits a new global symmetry. This is possible via the introduction of a quasi-dilaton scalar
field 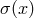 . The action for QMG is thus given by
. The action for QMG is thus given by
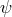 represent the matter fields,
represent the matter fields, 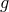 is the dynamical metric, and unless specified otherwise all indices
are raised and lowered with respect to
is the dynamical metric, and unless specified otherwise all indices
are raised and lowered with respect to 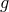 , and
, and  represents the scalar curvature with respect to
represents the scalar curvature with respect to 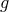 .
The Lagrangians
.
The Lagrangians 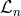 were expressed in (6.9* – 6.13*) or (6.14* – 6.18*) and the tensor
were expressed in (6.9* – 6.13*) or (6.14* – 6.18*) and the tensor 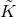 is given in terms of
the Stückelberg fields as
In the case of the QMG presented in [119*], there is no cosmological constant nor tadpole (
is given in terms of
the Stückelberg fields as
In the case of the QMG presented in [119*], there is no cosmological constant nor tadpole ( )
and
)
and 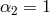 . This is a very special case of the generalized theory of massive gravity presented in [320], and
the proof for the absence of BD ghost thus goes through in the same way. Here again the presence of the
scalar field brings only minor modifications to the Hamiltonian analysis in the ADM language as presented
in Section 9.1, and so we do not reproduce the proof here. We simply note that the theory propagates six
degrees of freedom in four dimensions and is manifestly free of any ghost on flat space time provided that
. This is a very special case of the generalized theory of massive gravity presented in [320], and
the proof for the absence of BD ghost thus goes through in the same way. Here again the presence of the
scalar field brings only minor modifications to the Hamiltonian analysis in the ADM language as presented
in Section 9.1, and so we do not reproduce the proof here. We simply note that the theory propagates six
degrees of freedom in four dimensions and is manifestly free of any ghost on flat space time provided that
 . The key ingredient compared to mass-varying gravity or generalized massive gravity is the
presence of a global rescaling symmetry which is both a space-time and internal transformation [119*],
Notice that the matter action
. The key ingredient compared to mass-varying gravity or generalized massive gravity is the
presence of a global rescaling symmetry which is both a space-time and internal transformation [119*],
Notice that the matter action  breaks this symmetry, reason why it is called a
‘quasi-dilaton’.
breaks this symmetry, reason why it is called a
‘quasi-dilaton’.
An interesting feature of QMG is the fact that the decoupling limit leads to a bi-Galileon theory, one
Galileon being the helicity-0 mode presented in Section 8.3, and the other Galileon being the quasi-dilaton
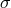 . Just as in massive gravity, there are no irrelevant operators arising at energy scale below
. Just as in massive gravity, there are no irrelevant operators arising at energy scale below 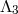 , and at
that scale the theory is given by
, and at
that scale the theory is given by
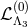 in the absence of the quasi-dilaton is given in (8.52*) and we
recall that
in the absence of the quasi-dilaton is given in (8.52*) and we
recall that 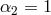 ,
, 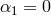 ,
, 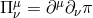 and the Lagrangians
and the Lagrangians 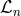 are expressed in (6.10*) – (6.13*) or
(6.15*) – (6.18*). We see emerging a bi-Galileon theory for
are expressed in (6.10*) – (6.13*) or
(6.15*) – (6.18*). We see emerging a bi-Galileon theory for 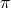 and
and 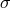 , and thus the decoupling limit is
manifestly ghost-free. We could then apply a similar argument as in Section 7.2.4 to infer the absence of
BD ghost for the full theory based on this decoupling limit. Up to integration by parts, the
Lagrangian (9.13*) is invariant under both independent Galilean transformation
, and thus the decoupling limit is
manifestly ghost-free. We could then apply a similar argument as in Section 7.2.4 to infer the absence of
BD ghost for the full theory based on this decoupling limit. Up to integration by parts, the
Lagrangian (9.13*) is invariant under both independent Galilean transformation 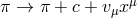 and
and
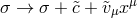 .
.
One of the relevance of this decoupling limit is that it makes the study of the Vainshtein mechanism more explicit. As we shall see in what follows (see Section 10.1), the Galileon interactions are crucial for the Vainshtein mechanism to work.
Note that in (9.13*), the interactions with the quasi-dilaton come in the combination
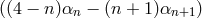 , while in
, while in 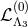 , the interactions between the helicity-0 and -2 modes come in
the combination
, the interactions between the helicity-0 and -2 modes come in
the combination 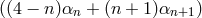 . This implies that in massive gravity, the interactions between
the helicity-2 and -0 mode disappear in the special case where
. This implies that in massive gravity, the interactions between
the helicity-2 and -0 mode disappear in the special case where  (this corresponds to the minimal model), and the Vainshtein mechanism is no longer active for
spherically symmetric sources (see Refs. [99*, 56*, 58*, 57*, 435*]). In the case of QMG, the interactions
with the quasi-dilaton survive in that specific case
(this corresponds to the minimal model), and the Vainshtein mechanism is no longer active for
spherically symmetric sources (see Refs. [99*, 56*, 58*, 57*, 435*]). In the case of QMG, the interactions
with the quasi-dilaton survive in that specific case 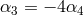 , and a Vainshtein mechanism
could still be feasible, although one might still need to consider non-asymptotically Minkowski
configurations.
, and a Vainshtein mechanism
could still be feasible, although one might still need to consider non-asymptotically Minkowski
configurations.
The cosmology of QMD was first discussed in [119*] where the existence of self-accelerating solutions was pointed out. This will be reviewed in the section on cosmology, see Section 12.5. We now turn to the extended version of QMG recently proposed in Ref. [127*].
9.2.2 Extended quasi-dilaton
Keeping the same philosophy as the quasi-dilaton in mind, a simple but yet powerful extension was proposed in Ref. [127*] and then further extended in [126*], leading to interesting phenomenology and stable self-accelerating solutions. The phenomenology of this model was then further explored in [45]. The stability of the extended quasi-dilaton theory of massive gravity was explored in [353] and was proven to be ghost-free in [406].
The key ingredient behind the extended quasi-dilaton theory of massive gravity (EMG) is to notice that
two most important properties of QMG namely the absence of BD ghost and the existence of a global
scaling symmetry are preserved if the covariantized reference metric is further generalized to include a
disformal contribution of the form 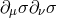 (such a contribution to the reference metric can
arise naturally from the brane-bending mode in higher dimensional braneworld models, see for
instance [157*]).
(such a contribution to the reference metric can
arise naturally from the brane-bending mode in higher dimensional braneworld models, see for
instance [157*]).
The action for EMG then takes the same form as in (9.10*) with the tensor 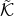 promoted to
promoted to
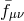 defined as
where
defined as
where 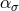 is a new coupling dimensionless constant (as mentioned in [127*], this coupling constant is
expected to enjoy a non-renormalization theorem in the decoupling limit, and thus to receive quantum
corrections which are always suppressed by at least
is a new coupling dimensionless constant (as mentioned in [127*], this coupling constant is
expected to enjoy a non-renormalization theorem in the decoupling limit, and thus to receive quantum
corrections which are always suppressed by at least 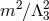 ). Furthermore, this action can be generalized
further by
). Furthermore, this action can be generalized
further by
- Considering different coupling constants for the
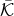 ’s entering in
’s entering in ![ℒ2[¯𝒦]](article1821x.gif) ,
, ![ℒ3 [𝒦¯]](article1822x.gif) and
and
![¯ ℒ4[𝒦 ]](article1823x.gif) .
.
- One can also introduce what would be a cosmological constant for the metric
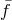 , namely a
new term of the form
, namely a
new term of the form 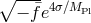 .
.
- General shift-symmetric Horndeski Lagrangians for the quasi-dilaton.
With these further generalizations, one can obtain self-accelerating solutions similarly as in the original QMG.
For these self-accelerating solutions, the coupling constant 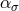 does not enter the background
equations of motion but plays a crucial role for the stability of the scalar perturbations on top of
these solutions. This is one of the benefits of this extended quasi-dilaton theory of massive
gravity.
does not enter the background
equations of motion but plays a crucial role for the stability of the scalar perturbations on top of
these solutions. This is one of the benefits of this extended quasi-dilaton theory of massive
gravity.
9.3 Partially massless
9.3.1 Motivations behind PM gravity
The multiple proofs for the absence of BD ghost presented in Section 7 ensures that the ghost-free theory of
massive gravity, (or dRGT) does not propagate more than five physical degrees of freedom in the graviton.
For a generic finite mass 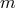 the theory propagates exactly five degrees of freedom as can be shown from a
linear analysis about a generic background. Yet, one can ask whether there exists special points
in parameter space where some of degrees of freedom decouple. General relativity, for which
the theory propagates exactly five degrees of freedom as can be shown from a
linear analysis about a generic background. Yet, one can ask whether there exists special points
in parameter space where some of degrees of freedom decouple. General relativity, for which
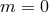 (and the other parameters
(and the other parameters 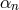 are finite) is one such example. In the massless limit of
massive gravity the two helicity-1 modes and the helicity-0 mode decouple from the helicity-2
mode and we thus recover the theory of a massless spin-2 field corresponding to GR, and three
decoupled degrees of freedom. The decoupling of the helicity-0 mode occurs via the Vainshtein
mechanism24
as we shall see in Section 10.1.
are finite) is one such example. In the massless limit of
massive gravity the two helicity-1 modes and the helicity-0 mode decouple from the helicity-2
mode and we thus recover the theory of a massless spin-2 field corresponding to GR, and three
decoupled degrees of freedom. The decoupling of the helicity-0 mode occurs via the Vainshtein
mechanism24
as we shall see in Section 10.1.
As seen in Section 8.3.6, when considering massive gravity on de Sitter as a reference metric, if the
graviton mass is precisely  , the helicity-0 mode disappears linearly as can be seen from the
linearized Lagrangian (8.62*). The same occurs in any dimension when the graviton mass is tied to the de
Sitter curvature by the relation
, the helicity-0 mode disappears linearly as can be seen from the
linearized Lagrangian (8.62*). The same occurs in any dimension when the graviton mass is tied to the de
Sitter curvature by the relation 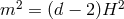 . This special case is another point in parameter space
where the helicity-0 mode could be decoupled, corresponding to a partially massless (PM) theory of gravity
as first pointed out by Deser and Waldron [190*, 189*, 188*], (see also [500] for partially massless higher spin,
and [450] for related studies).
. This special case is another point in parameter space
where the helicity-0 mode could be decoupled, corresponding to a partially massless (PM) theory of gravity
as first pointed out by Deser and Waldron [190*, 189*, 188*], (see also [500] for partially massless higher spin,
and [450] for related studies).
The absence of helicity-0 mode at the linearized level in PM is tied to the existence of a new scalar
gauge symmetry at the linearized level when 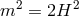 (or
(or 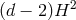 in arbitrary dimensions), which
is responsible for making the helicity-0 mode unphysical. Indeed the action (8.62*) is invariant
under a special combination of a linearized diff and a conformal transformation [190, 189, 188],
in arbitrary dimensions), which
is responsible for making the helicity-0 mode unphysical. Indeed the action (8.62*) is invariant
under a special combination of a linearized diff and a conformal transformation [190, 189, 188],
- It would protect the structure of the potential.
- In the PM limit of massive gravity, the helicity-0 mode fully decouples from the helicity-2 mode and hence from external matter. As a consequence, there is no Vainshtein mechanism that decouples the helicity-0 mode in the PM limit of massive gravity unlike in the massless limit. Rather, the helicity-0 mode simply decouples without invoking any strong coupling effects and the theoretical and observational luggage that goes with it.
- Last but not least, in PM gravity the symmetry underlying the theory is not diffeomorphism invariance but rather the one pointed out in (9.16*). This means that in PM gravity, an arbitrary cosmological constant does not satisfy the symmetry (unlike in GR). Rather, the value of the cosmological constant is fixed by the gauge symmetry and is proportional to the graviton mass. As we shall see in Section 10.3 the graviton mass does not receive large quantum corrections (it is technically natural to set to small values). So, if a PM theory of gravity existed it would have the potential to tackle the cosmological constant problem.
Crucially, breaking of covariance implies that matter is no longer covariantly conserved. Instead the failure of energy conservation is proportional to the graviton mass,
which in practise is extremely small.It is worth emphasizing that if a PM theory of gravity existed, it would be distinct from the minimal model of massive gravity where the non-linear interactions between the helicity-0 and -2 modes vanish in the decoupling limit but the helicity-0 mode is still fully present. PM gravity is also distinct from some specific branches of solutions found in cosmology (see Section 12) on top of which the helicity-0 mode disappears. If a PM theory of gravity exists the helicity-0 mode would be fully absent of the whole theory and not only for some specific branches of solutions.
9.3.2 The search for a PM theory of gravity
A candidate for PM gravity:
The previous considerations represent some strong motivations for finding a fully fledged theory of PM gravity (i.e., beyond the linearized theory) and there has been many studies to find a non-linear realization of the PM symmetry. So far all these studies have in common to keep the kinetic term for gravity unchanged (i.e., keeping the standard Einstein–Hilbert action, with a potential generalization to the Lovelock invariants [298*]).
Under this assumption, it was shown in [501, 330], that while the linear level theory admits a symmetry
in any dimensions, at the cubic level the PM symmetry only exists in  spacetime dimensions, which
could make the theory even more attractive. It was also pointed out in [191] that in four dimensions the
theory is conformally invariant. Interestingly, the restriction to four dimensions can be lifted in bi-gravity by
including the Lovelock invariants [298*].
spacetime dimensions, which
could make the theory even more attractive. It was also pointed out in [191] that in four dimensions the
theory is conformally invariant. Interestingly, the restriction to four dimensions can be lifted in bi-gravity by
including the Lovelock invariants [298*].
From the analysis in Section 8.3.6 (see Ref. [154*]) one can see that the helicity-0 mode entirely
disappears from the decoupling limit of ghost-free massive gravity, if one ignores the vectors and sets the
parameters of the theory to 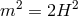 ,
, 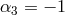 and
and 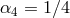 in four dimensions. The ghost-free
theory of massive gravity with these parameters is thus a natural candidate for the PM theory of gravity.
Following this analysis, it was also shown that bi-gravity with the same parameters for the
interactions between the two metrics satisfies similar properties [301*]. Furthermore, it was also
shown in [147*] that the potential has to follow the same structure as that of ghost-free massive
gravity to have a chance of being an acceptable candidate for PM gravity. In bi-gravity the same
parameters as for massive gravity were considered as also being the natural candidate [301*], in
addition of course to other parameters that vanish in the massive gravity limit (to make a fair
comparison once needs to take the massive gravity limit of bi-gravity with care as was shown
in [301*]).
in four dimensions. The ghost-free
theory of massive gravity with these parameters is thus a natural candidate for the PM theory of gravity.
Following this analysis, it was also shown that bi-gravity with the same parameters for the
interactions between the two metrics satisfies similar properties [301*]. Furthermore, it was also
shown in [147*] that the potential has to follow the same structure as that of ghost-free massive
gravity to have a chance of being an acceptable candidate for PM gravity. In bi-gravity the same
parameters as for massive gravity were considered as also being the natural candidate [301*], in
addition of course to other parameters that vanish in the massive gravity limit (to make a fair
comparison once needs to take the massive gravity limit of bi-gravity with care as was shown
in [301*]).
Re-appearance of the Helicity-0 mode:
Unfortunately, when analyzing the interactions with the vector fields, it is clear from the decoupling
limit (8.52*) that the helicity-0 mode reappears non-linearly through their couplings with the vector fields.
These never cancel, not even in four dimensions and for no parameters of theory. So rather than being free
from the helicity-0 mode, massive gravity with 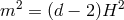 has an infinitely strongly coupled
helicity-0 mode and is thus a sick theory. The absence of the helicity-0 mode is simple artefact of the linear
theory.
has an infinitely strongly coupled
helicity-0 mode and is thus a sick theory. The absence of the helicity-0 mode is simple artefact of the linear
theory.
As a result we can thus deduce that there is no theory of PM gravity. This result is consistent with many independent studies performed in the literature (see Refs. [185, 147, 181, 194]).
Relaxing the assumptions:
- One assumption behind this result is the form of the kinetic term for the helicity-2 mode, which is kept to the be Einstein–Hilbert term as in GR. A few studies have considered a generalization of that kinetic term to diffeomorphism-breaking ones [231, 310] however further analysis [339, 153] have shown that such interactions always lead to ghosts non-perturbatively. See Section 5.6 for further details.
- Another potential way out is to consider the embedding of PM within bi-gravity or multi-gravity. Since bi-gravity is massive gravity and a decoupled massless spin-2 field in some limit it is unclear how bi-gravity could evade the results obtained in massive gravity but this approach has been explored in [301, 298, 299*, 184*]. A perturbative relation between bi-gravity and conformal gravity was derived at the level of the equations of motion in Ref. [299] (unlike claimed in [184]).
- The other assumptions are locality and Lorentz-invariance. It is well known that Lorentz-breaking theories of massive gravity can excite fewer than five degrees of freedom. This avenue is explored in Section 14.
To summarize there is to date no known non-linear PM symmetry which could project out the helicity-0 mode of the graviton while keeping the helicity-2 mode massive in a local and Lorentz invariant way.

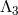 -decoupling
limit of bi-gravity
-decoupling
limit of bi-gravity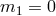
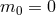 )
)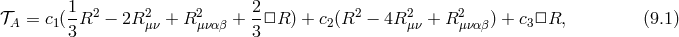
![2 ∫ ( 2 ∑4 ℒMass−Varying = M-Pl d4x√ −-g R + m--(ψ-) αnℒn [𝒦] (9.2 ) 2 2 n=0 ) 1-μν − 2g ∂μ ψ∂νψ − W (ψ) ,](article1760x.gif)
![2 ∫ [ 4 ℒ = M-Pl d4x√ −-g Ω (ψ )R + 1∑ α (ψ )ℒ [𝒦 ] (9.3 ) Generalized MG 2 A 2 n A n ] n=0 − 1gμν∂ ψ ∂ ψA − W (ψ ) . 2 μ A ν A](article1766x.gif)
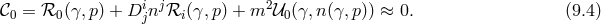
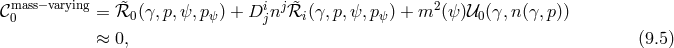
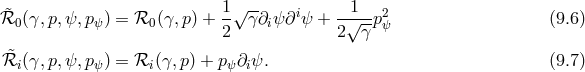
![∂m2 (ψ )[ 𝒩¯ ] 𝒞&tidle;2 = 𝒞2 + -------- 𝒰0∂iψ(𝒩¯ni + 𝒩¯i) + √--𝒰1p ψ + 𝒩¯∂iψDiknk ∂ψ γ ≈ 0. (9.8 )](article1773x.gif)
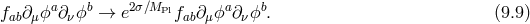
![∫ 4 M-2Pl 4 √ ---[ -ω--- 2 m2-∑ &tidle; ] SQMG = 2 d x − g R − 2M 2 (∂σ) + 2 αn ℒn[𝒦 [g,η ]] (9.10 ) ∫ Pl n=0 4 √ --- + d x − gℒmatter(g,ψ),](article1782x.gif)
![∘ --------------- &tidle;𝒦μ[g,η] = δμ − eσ∕MPl gμα∂α ϕa∂νϕbηab. (9.11 ) ν ν](article1790x.gif)
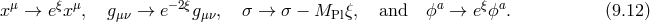
![∑4 ℒ(QMG )= ℒ(0)− ω(∂σ )2 + 1σ (4-−-n-)αn-−-(n-+-1)αn+1-ℒn[Π ], (9.13 ) Λ3 Λ3 2 2 n=1 Λ3(3n−1)](article1798x.gif)